
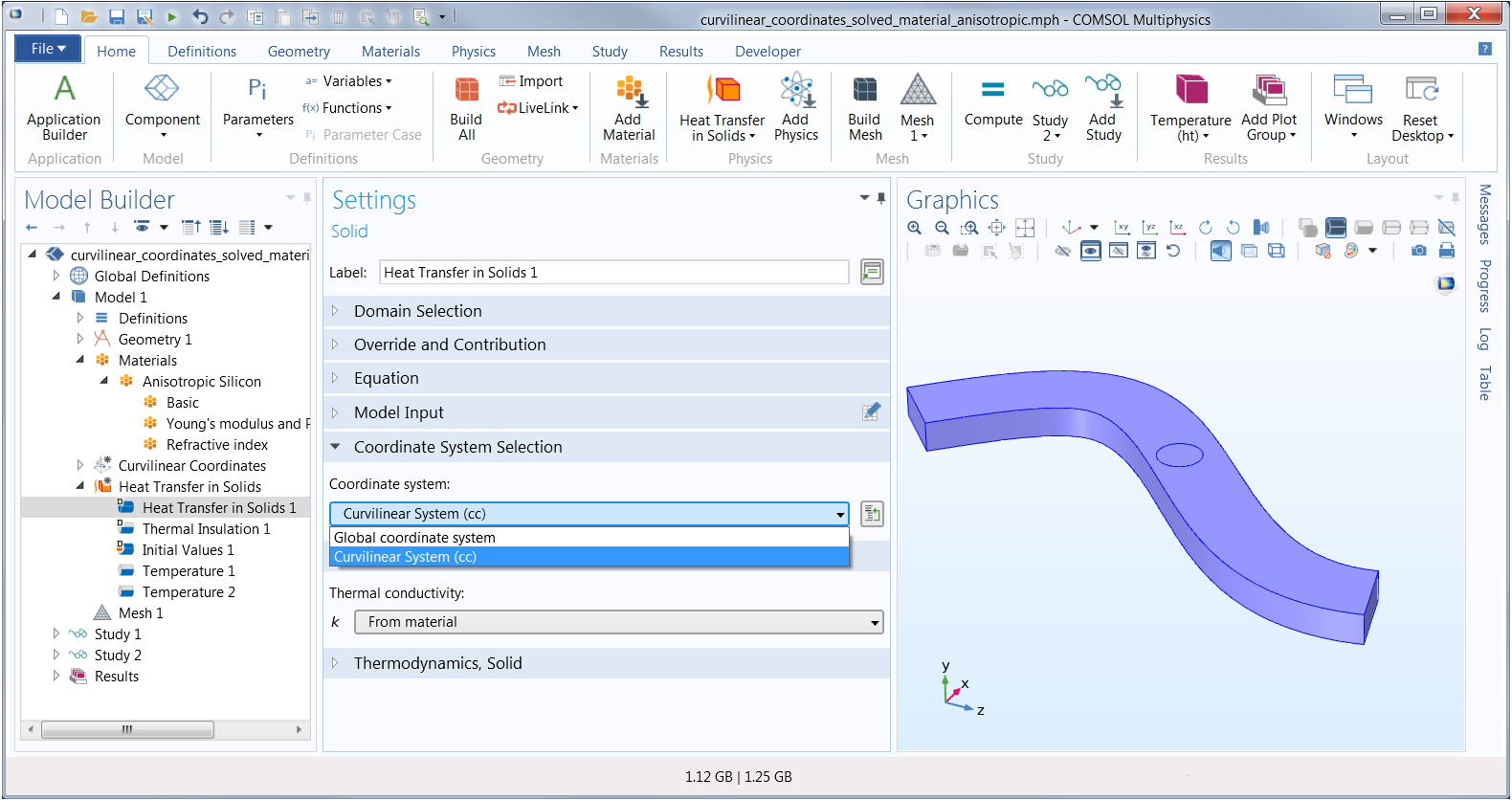
K ∈ T h, as being the restrictions to the finite elements K of the function v ∈ X h, just as if v were an “ordinary” function defined over the set It is also a usual practice to consider the functions v K. Nevertheless, by virtue of assumption (2.3.22), it is customary to say that the “functions” in the space X h are at least “continuous at all nodes common to adjacent finite elements” (the inclusions P K ⊂ C 0( K), K ∈ T h, hold in practice). Ω ¯, since it need not have a unique definition along faces common to adjacent finite elements. It is thus realized that an element v ∈ X h is not in general a “function” defined over the set Which is called the set of degrees of freedom of the finite element space. Various justifications are possible, we will use a Galerkin method and, we will consider a regularization.
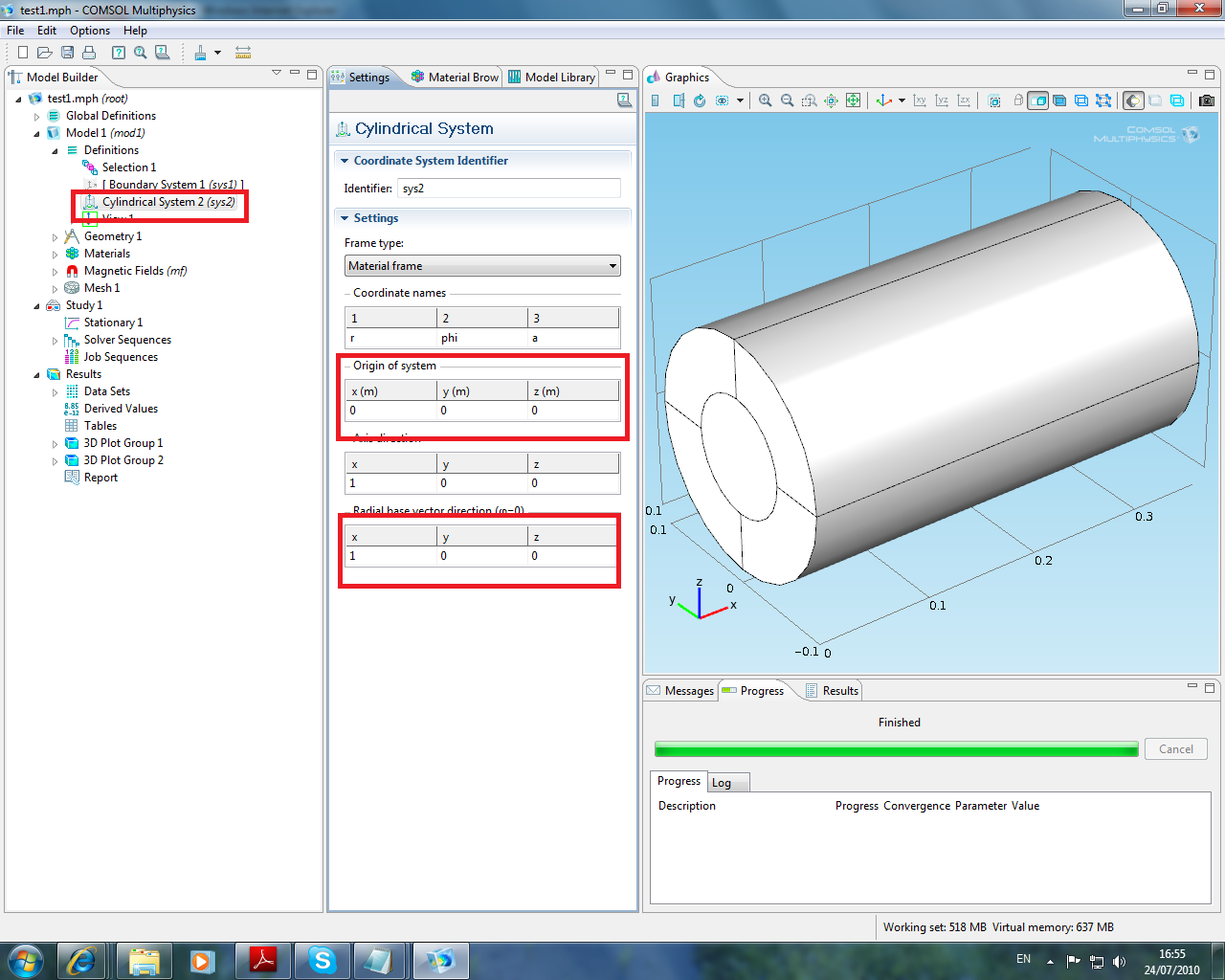
In this chapter, the computation of derivatives is performed in a formal sense only. Depending on how we choose to determine the family of problems for the invariant embedding, multiple factorizations are possible.

After defining our model problem and its functional framework, we will show how spatial invariant embedding leads to a system of decoupled evolution equations equivalent to the boundary value problem. However, from the point of view of solving elliptic problems, this geometry is a special case, and the generalization of the spatial invariant embedding method to other shapes of domain will be studied.

We will that this geometry arises naturally when we make an analogy with the optimal control problem for parabolic systems. The operator for this problem is naturally the Laplacian and a cylindrical domain is assumed. In this chapter, we introduce a “model problem”, denoted by ( P 0 ), of an elliptic boundary value problem, which we will use to describe the use of spatial invariant embedding and the factorized forms that follow from it. Results were evaluated after the lift and drag reached a pseudo-periodic state. The solution was marched forward in time using the RK54 approach and, at each time-step, the inviscid and viscous numerical fluxes were computed using a Rusanov-type Riemann solver. The simulations were run using two different tetrahedral ESFR schemes: one with c = 0 designed to recover a collocated nodal DG scheme and another with c = c + where c + is chosen to maximise the permissible explicit time step. The angle of entry for the incoming flow was set as 4 degree in order to simulate a 4 degree angle of attack. The Reynolds number was taken to be Re = 10, 000 with the incoming flow having a velocity of Mach M = 0.2. The domain was then meshed with 711,332 quadratically curved tetrahedral elements. Periodic boundary conditions were prescribed on the front and back of the cylindrical domain, characteristic boundary conditions were prescribed on the sides of the domain, and adiabatic wall boundary conditions were prescribed on the surface of the wing-section. The numerical experiments of Williams on the SD7003 geometry were performed on a cylindrical domain formed by extruding a circular domain (of radius 20 c) by 0.2 c in the z direction where c is the chord. The SD7003 geometry is that of a low Reynolds number Selig/Donovan airfoil that has a maximum thickness of 9.2 % at 30.9 % chord, and a maximum camber of 1.2 % at 38.3 % chord. (2016) and Williams (2013) have used FR schemes to simulate flow over an SD7003 infinite wing at 4 degrees angle of attack. Jameson, in Handbook of Numerical Analysis, 2016 6.3 Flow Over an SD7003 Wingīoth Vermeire et al. A particular situation is studied where the domain is star shaped and the subdomains are obtained from the initial domain by a homothety.į.D. Further, we turn to the general case where the surfaces of the family have no edge. Then, the case of subdomains limited by a plane surface but with a non-orthogonal displacement is studied. In the first step, we study the situation where there is a transformation preserving orthogonality that allows us to reduce the problem to the cylindrical case. In the non-cylindrical case, we need to define, on the one hand, a family of surfaces continuously sweeping over the initial domain and which limit the subdomains, and, on the other hand, a displacement field between these surfaces. The cylindrical case is especially simple as the subdomains are just sub-cylinders and an infinitesimal variation of the domain is achieved by moving the limiting boundary by an infinitesimal amount in the orthogonal direction. In this chapter, we want to show, without completeness, that the success of the invariant embedding method for the factorization of elliptic boundary value problems is not bound to cylindrical domains. Ramos, in Factorization of Boundary Value Problems Using the Invariant Embedding Method, 2016 Abstract:


 0 kommentar(er)
0 kommentar(er)
